Cross+A Logiikkapelien esimerkkejä

• Slitherlink
• Lampaat ja sudet
• Battleships
• Digital Battleships
• Retrograde Battleships
• Fillomino
• No Rectangles Fillomino
• Only Rectangles Fillomino
• No 2 x 2 Squares Fillomino
• Non-Consecutive Fillomino
• Consecutive Fillomino
• No Row/Column Repeats Fillomino
• All Odds Fillomino
• All Evens Fillomino
• Nurikabe
• Line Nurikabe
• Pairs Nurikabe
• Skyscrapers
• Sum Skyscrapers
• Tapa
• Tapa [Line]
• No Squares Tapa
• Equal Tapa
• B&W Tapa
• Tapa Islands
• Pata
• Tapa Balance
• Tapa Row
• Tapa 1-n
• Dissected Tapa
• Tapa [Diagonal Neighbors]
• Corral
• Inside/Outside Corral
• Arukone
• Arukone³
• Easy as ABC
• Not as Easy as ABC
• Ichimaga
• Crossing Ichimaga
• Magnetic Ichimaga
• Snake
• Multiple Snakes
• Toroidal Snake
• Minesweeper
• Double Minesweeper
• Mirukuti
• Milk Tease
• Light Up
• Mirror Akari
• Tren
• Tren+
• Ghost Tren
• Mubunanba
• Mubunanba+
• Yajilin
• Regional Yajilin
• LITS
• Double LITS
• Kapama
• Sunglasses
• Makaro
• Masakuchi

Lampaat ja sudet ("Sheep and Wolves") on Slitherlink-logiikkapelin laji: numerojen 0-3 lisäksi tehtävässä on myös mustia ympyröitä (lampaat) ja ristejä (sudet). Tehtävän ratkaistua "lampaiden" tulee olla säätöpiirissä (tarhassa) entä susien tarhan ulkopuolella.

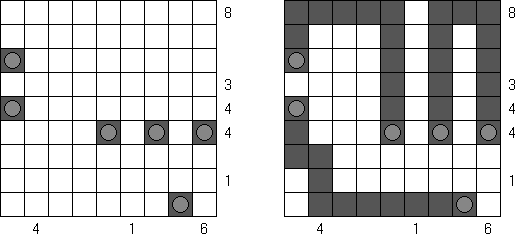
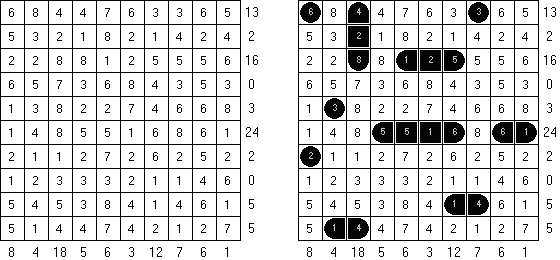
In Digital Battleships all cells of the grid contain numbers. The values on the right and bottom edges of the grid reveal the sum of the numbers in each of the ship pieces that appear in each respective row or column.

Retrograde Battleships ("Reverse Battleships") contains all segments of the ships in the grid. The aim is to find the correct locations of the ships. No ship may touch another, even diagonally.

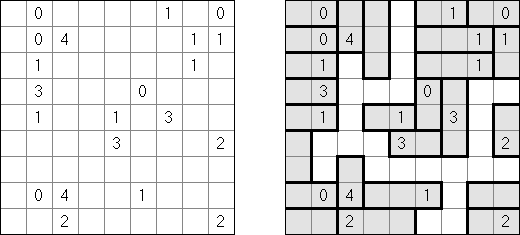
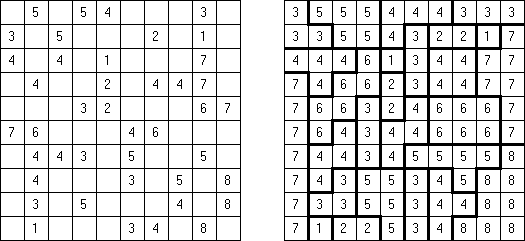
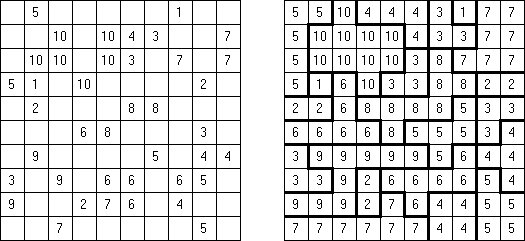
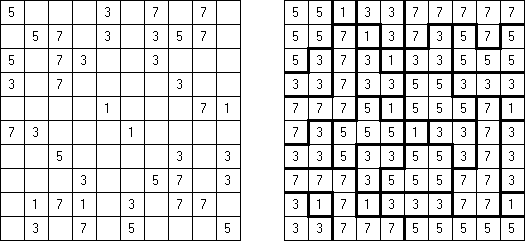
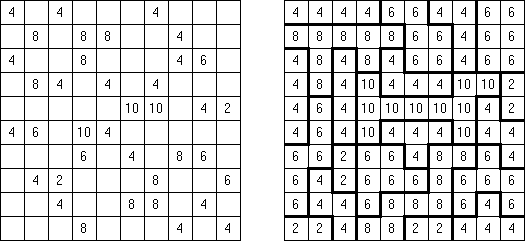
No Rectangles Fillomino sisältää yhden lisäsäännön: ei mikään yksikkö voi olla suorakulmion tai neliön muotoinen.

Only Rectangles Fillomino: kaikkien yksikköjen tulee olla suorakulmion tai neliön muotoisia.

No 2 x 2 Squares Fillomino: ruudukkoon ei saa sisältyä yhtäkään 2x2-ruudukkoa, jossa on samoja numeroja.

Non-Consecutive Fillomino: kahden vaara- tai pystysuorassa toisiinsa koskevan yksikön ruudut eivät saa erota toisistaan ykkösellä.

Consecutive Fillomino: jokaisen yksikön tulee koskea vaaka- tai pystysuorassa vähintään yhteen muuhun yksikköön, jonka koko eroaa sen koosta vähintään yhdellä ruudulla.

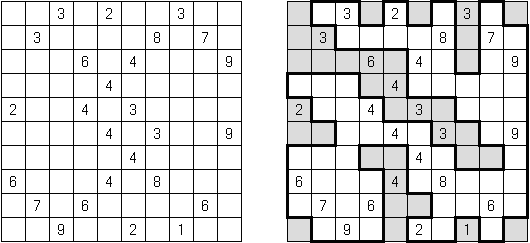
No Row/Column Repeats Fillomino ("Deadomino"): samaan riviin tai sarakkeeseen sisältyvät kaikkien samojen numerojen tulee sisältyä samaan yksikköön. Esimerkiksi, jos rivissä on kaksi viitosta, niin ne molemmat sisältyvät samaan yksikköön.

All Odds Fillomino: kaikkien yksikköjen koot ovat parittomia.

All Evens Fillomino: kaikkien yksikköjen koot ovat parillisia.

Line Nurikabe: a grid cannot contain five consecutive black cells in a row or column (2 x 2 cell area can be all black).

Pairs Nurikabe eroaa tavallisesta Nurikabe-pelista sillä tavalla, että jokaiseen "saareen" sisältyy kaksi lukua, joiden summa on sama kuin "saaren" pinta-ala.

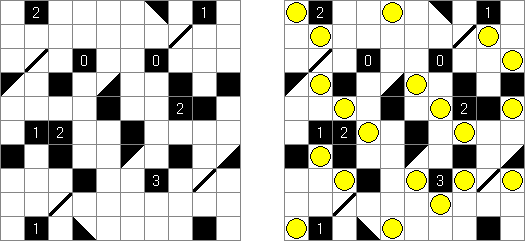
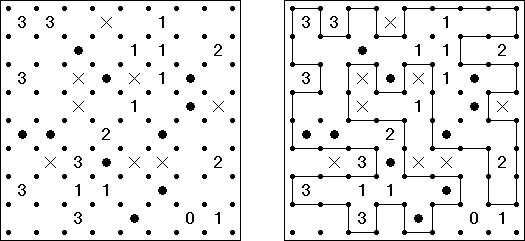
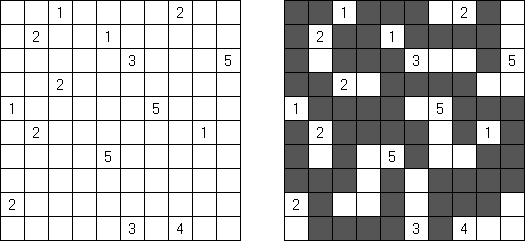
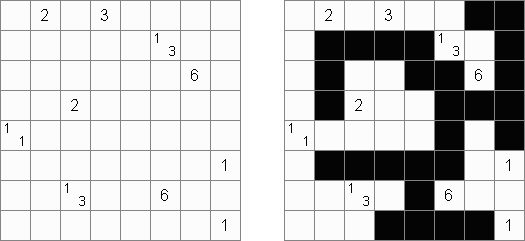
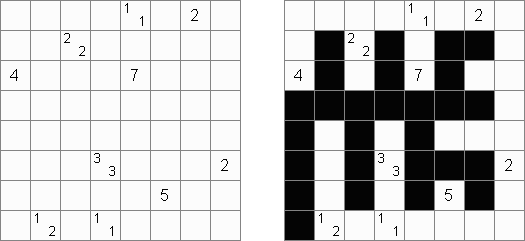
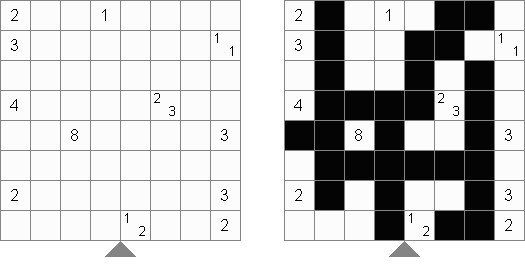
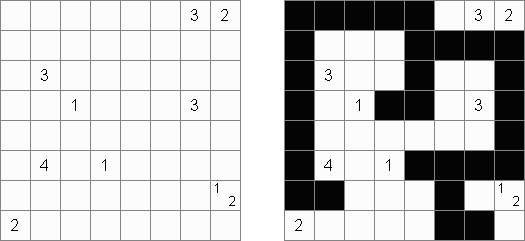
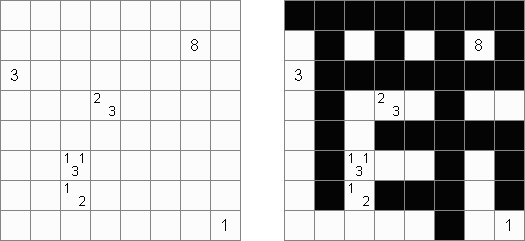
Sum Skyscrapers eroaa tavallisesta Skyscrapers-pelista sillä tavalla, että, marginaaleissa olevat luvut eivät kerro näkyvissä olevien talojen lukumäärästä, vaan niiden talojen kaikkien kerrosten summasta.

Olemassa on monta Tapa-pelin muunnosta. Cross+A pystyy ratkaisemaan jotkin niistä.

Tapa-pelin [Line]-versiossa käytössä on lisäohje: mustien ruutujen muodostama vaaka- tai pystysuoran rivi voi olla enintään kolme ruutua pitkä.

No Squares Tapa: ruudukossa ei saa olla yhtäkään 2 x 2 ruutua, joka koostuu vain valkoisista ruuduista.

Equal Tapa: valkoisten ruutujen lukumäärä on sama kuin mustien ruutujen (paitsi lukuruutuja).

B&W Tapa:

• Kaikki valkoiset ruudut muodostavat yhtenäisen alueen.
• Olemassa ei saa olla yhtäkään 2 x 2 ruutua, joka koostuu ainoastaan valkoisista tai mustista ruuduista.
• Lukuruudut pidetään valkoisina ruutuina.

Tapa Islands:

• Valkoisista ruuduista muodostuvat erilliset alueet ("saaret").
• Jokainen valkoinen alue voi sisältää enintään yhden lukuruudun.
• Yksi ruuduissa olevista luvuista on sama kuin "saaren" alue.
• Olemassa voi olla myös valkoisia alueita ilman lukuruutuja.

Pata:

• Luvut tarkoittavat valkoisista ruuduista muodostuvia yhtenäisiä alueita.
• Eri valkoisten ruutujen alueiden välissä on vähintään yksi musta ruutu.

Tapa Balance: ruudukon vasemmalla puolella olevien mustien ruutujen lukumäärä on sama kuin ruudukon oikealla puolella. Valkoisia ruutuja eikä lukuruutuja ei huomioida.

Tapa Row: rivin kaikkien lukujen summa on sama kuin saman rivin kaikkien mustien ruutujen lukumäärä.

Tapa 1-n: kaikissa riveissä ja sarakkeissa olevat mustien ruutujen lukumäärät ovat erilaisia.

Dissected Tapa: mustat ja valkoiset ruudut muodostavat kaksi yhteensopivaa kuviota. Kahta kuviota pidetään yhteensopivina kun ne ovat samoja toistensa päälle pantaessa.

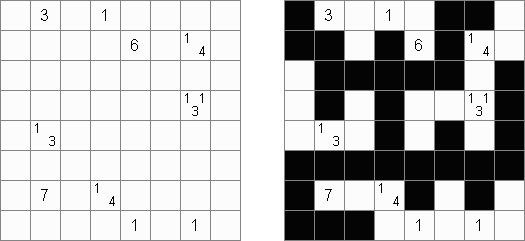
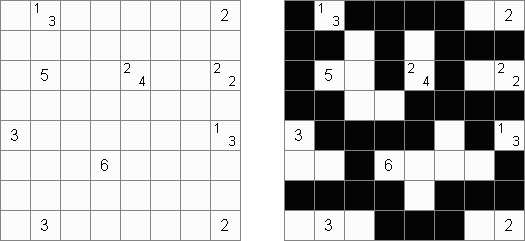
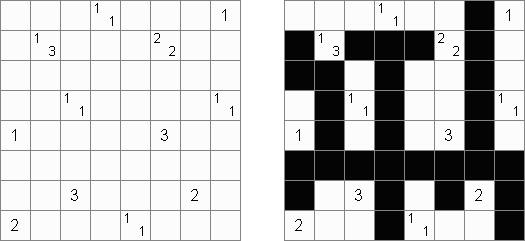
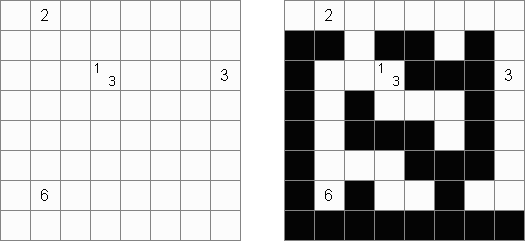
Tapa [Diagonal Neighbors]: jokaisen mustan ruudun vieressä tulee olla vähintään yksi musta ruutu, johon se koskee vinottain.

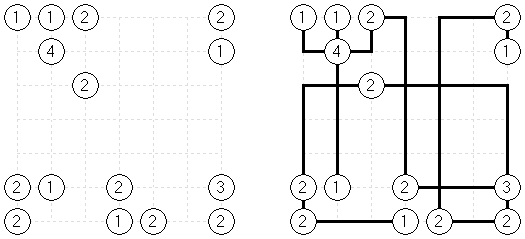
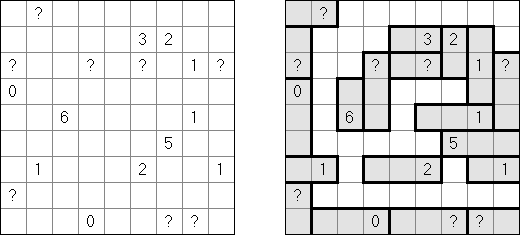
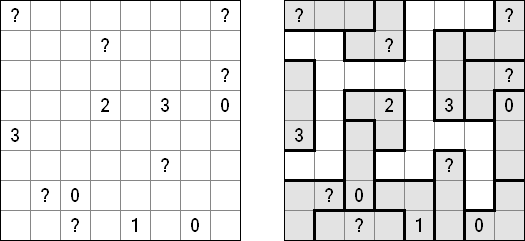
Inside/Outside Corral on Corral-logiikkapelin laji. Numerot voivat sisältyä suljetun linja sekä sisä-, että ulkopuolelle. Molemmissa tapauksissa numero tarkoittaa "näkyvien" ruutujen määrää oma ruutu mukaan luettuna.

Arukone³:

• Every line must not cover 2 x 2 area.
• All cells in the grid must be filled.

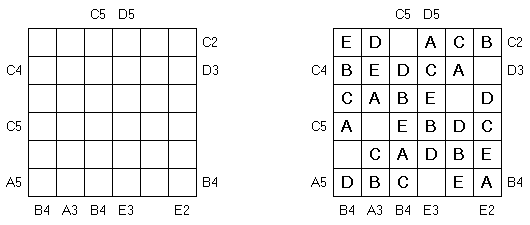
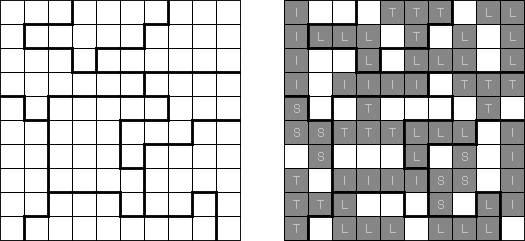
Not as Easy as ABC is a variation of Easy as ABC puzzle. The goal is to fill in the first letters of the alphabet on every row and every column exactly once. One cell in every row and every column remains empty. Letters and numbers on the outside indicate at what position you come across this letter when looking from that side (e.g. C₂ means the C is the second letter encountered when looking from that side).

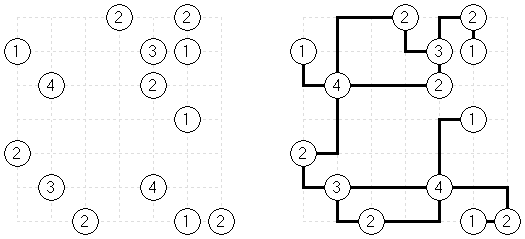
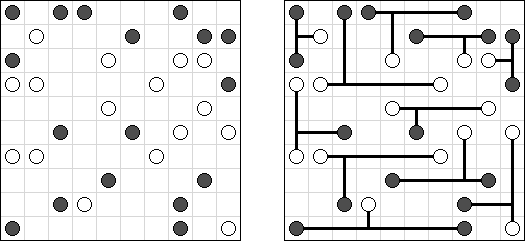
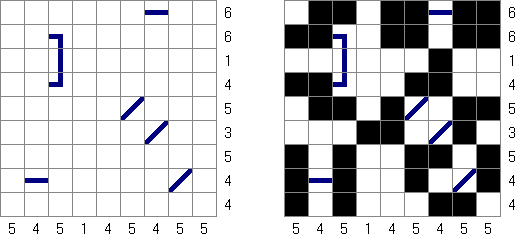
Crossing Ichimaga: the lines may cross other lines; the lines cannot change direction at the point of intersection.

Magnetic Ichimaga ("Jishaku-Ichimaga"): the circles with the same digits cannot be connected.

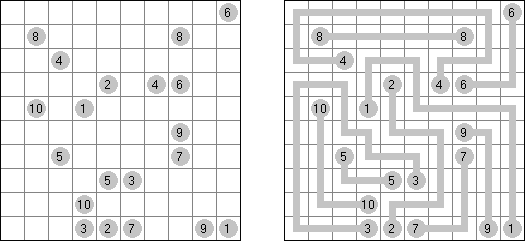
Multiple Snakes: a grid contains multiple snakes. Head and tail of all snakes are given. Different snakes do not touch each other, even diagonally.

Toroidal Snake: a grid wraps around itself. A snake can go from one edge to another.

Double Minesweeper: place mines into each empty cell in the grid, at most two mines per cell.

Milk Tease is a variation of Mirukuti ("Milk-T") puzzle. T-shaped line may connect:

• one black circle and two white circles;
• one white circle and two black circles.

Two circles of the same color must be connected by the straight-line segment of the T-shaped line.

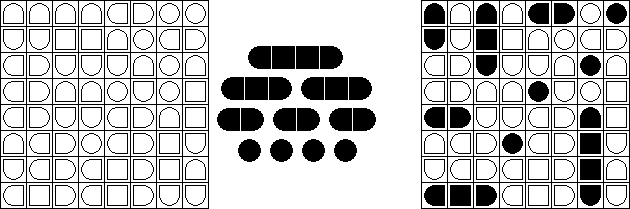
Mirror Akari ("!irakAkari!"): a square or rectangular grid contains diagonal walls (black triangles) with mirrors.

• No two bulbs shine on each other, even by reflected light. A bulb cannot shine on itself.
• If a cell contains a diagonal wall ("mirror"), it should be lit.
• If a cell contains a diagonal line ("two-sided mirror"), both sides of a mirror should be lit.
• A cell with a mirror cannot contain a bulb.

Tren+:

• Each block contains exactly one number or a question sign (unknown number), indicating the amount of its possible movements.
• All cells that are not part of any block must be connected horizontally or vertically.

Ghost Tren:

• Blocks can also be placed without any numbers, with no restrictions on their ability to move.
• All cells that are not part of any block must be connected horizontally or vertically.

Mubunanba+:

• Each block contains exactly one digit or a question sign (unknown digit), indicating the number of possible directions to move a block.
• All cells that are not part of any block must be connected horizontally or vertically.

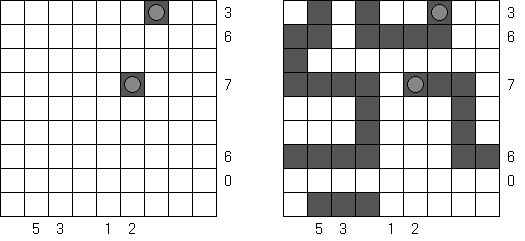
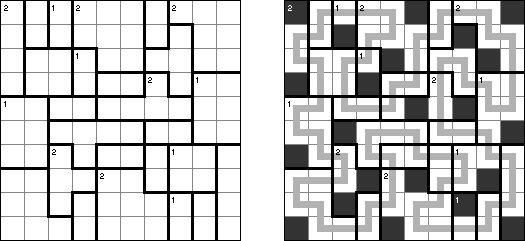
Regional Yajilin (also known as "Yajilin (Regions)") is a square or rectangular grid divided into regions. The aim is to blacken some cells and to draw a single non-intersecting loop through all the white cells. A number in a region indicates the number of black cells in that region. A region without a number can contain any amount of black cells. No two black cells can share a border. The loop may visit numbered cells; numbered cells can be blackened.

Double LITS has one difference from the classic variant of the puzzle: each region must contain two tetrominoes. These two tetrominoes within a region cannot touch each other horizontally or vertically (only diagonally); they can be the same or different shapes.

Sunglasses is a logic puzzle. A rectangular or square grid contains lines ("bridges") in some cells. The goal is to blacken some cells to create pairs of figures (twin shapes). Twin shapes ("lenses") are symmetrical with respect to a bridge. Two lenses may not share an edge. Cells with bridges can not be blacken. Numbers outside the grid show the number of black cells in a corresponding row or column.

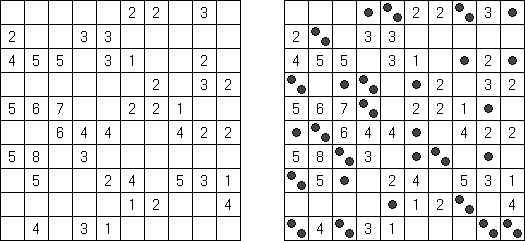
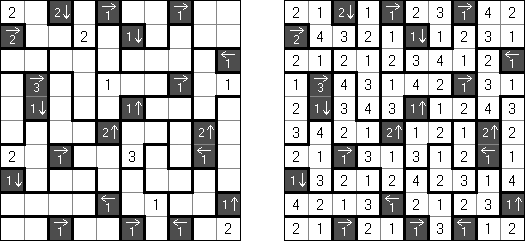
Masakuchi is a logic puzzle published by Nikoli. A rectangular or square grid is divided into regions. Each region must be filled with each of the digits from 1 to the number of cells in the region. When two numbers are orthogonally adjacent across a region boundary, the numbers must be different. The grid may contain black cells with arrows and numbers: the arrow points at the greatest number among the four cells around (up, under, left, right) the black cell. The number in the black cell shows the difference between the greatest number and the second highest number in all orthogonally adjacent cells around the black cell.